|
Here is the same graph but using an inductor instead of a capacitor.
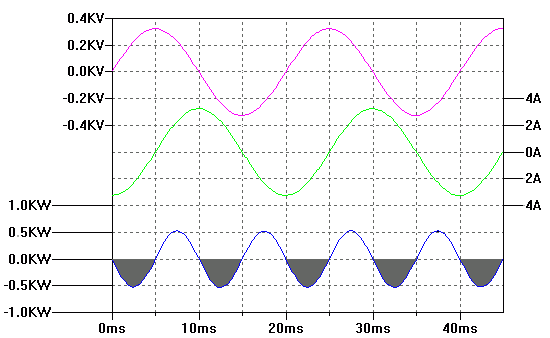
This is very similar to the graph of the capacitor. The difference is that this time the current is lagging the voltage by 90 degrees instead of leading the voltage as it was with a capacitor.
But as far as the power is concerned it has exactly the same effect. The load (the inductor this time) stores power on one quarter cycle, then returns it to the source on the next quarter cycle. And again, for the technically minded, the inductor stores the energy in the magnetic field as opposed to the electric field as is the case in a capacior.
Now why is this so important? Why is it so relevant?
Well, if we say that Apparent Power is VA (which it is) and Real Power is Watts what we are really interested in is how much power our load can use to do useful work, whether that be heating a room, lighting something up or making a machine move.
The "real power" (Watts) is what really matters. The apparent power (VA) could include power returned to the source which actually does no useful work for us.
We have seen above how a capacitor or an inductor can cause the same current to flow but without actually doing any useful work. The Real Power is zero. But the generator, inverter, cabling, fuses etc all have to be sized to handle the same current, even though nothing useful is being done with it. If you have another look over the 3 graphs shown so far you will see that the current was identical in each graph.
"Apparent Power" is the volts multiplied by the amps, which as you now realise is useless because it gives no indication of actually how much useful work is being done with it. However it does have its use in that it shows that although no useful work is being done, the cabling, fuses, generator etc all have to be able to provide and handle the relevant current.
In all the three graphs just covered the "Apparent Power" is 230 volts * 2.3 amps = 530VA. But only in the case of the resistive load did it do any useful work (ie heating up the resistor). In both the other two cases the "Apparent Power" was still 530 VA but no useful work was done whatsoever. Your generator, inverter or whatever would still have to be rated above 530VA even though nothing was being achieved by it!
So what's this Power Factor thing?
The Power Factor is, as previously mentioned, a number between zero and 1 that is multiplied by the VA to give the Real Power.
But where does this number come from?
Well in this regard (ie that of phase shift between the applied voltage and the current drawn) the Power Factor is the cosine of the phase angle between the voltage and the current.
In the case of the resistive load the current and voltage were perfectly in phase. There was zero degrees phase shift. The cosine of 0 = 1. So Apparent Power of 530VA * 1 = 530 Watts (note we can now corectly use the term "Watts").
In the case of the capacitor and the inductor the phase shift was 90 degrees in both cases. Cosine 90 = 0 so 530VA * 0 = 0 Watts (again note we can now legitimately use the term "Watts")
And now on to a "halfway house" case...........
|
Page last updated 02/04/2008.
Website best viewed on a computer of some sort.