|
You'll be pleased to know that we're now almost finished on the matter of Power Factor, Apparent Power and Real Power.
Take a look at the following graph.
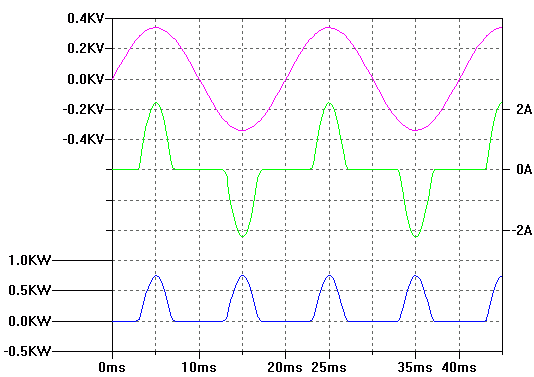
Here we have the same 230 volt AC power but the traces below it are very different to the ones we looked at earlier.
In the previous cases we simply had current drawn with the same waveshape (a sinewave) as that of the supplied 230 volts but with a phase lead or phase lag causing the varying Power Factor. In this case the current is not drawn in the same sinusoidal waveform. It is highly distorted.
Now whilst this does not lead to power being returned to the source as it did in the previous examples it does have its own different problems.
Look at the current waveform. Most of the time this load isn't actually drawing any current. It draws its current in short peaks.
Now if you think about this it means that, had the load drawn its current for more of the time, it would have had to draw less for the time it was drawing current in order to achieve the same overall power.
You'll be pleased to know that we don't even need to go into the maths behind this in order to understand it. Look at the power trace at the bottom. It is clear from this that, although it peaks at about 700 VA, because there is zero power for much of the time, the actual average power is very low indeed. In this case probably around 200 VA.
Now with a 230 volt supply, if the current was drawn sinusoidally (as in the first examples in this section) it would only need to draw 200VA/230V=0.87Amps RMS. This would have a peak current of 1.23Amps.
Yet look at the current waveform in the graph. It peaks at about 2.2 amps. Almost twice as much.
This means that the power source of a generator, invertor or isolation transformer has to be able to provide almost twice as much peak current as it would if the load was drawn sinusoidally.
In this case a Real reading power meter would show the Real Power as being about 200 Watts (which it is) but there is no way a 200 Watt inverter would be able to power it because a 200 Watt inverter would not have a high enough peak current rating to achieve this. A 200 Watt inverter only needs a peak current capability of about 0.9 Amps in order to achieve 200 Watts output rating. But in this case it would need a peak current output capability of 2.3 Amps. That equates to 1.63 Amps RMS and 1.63A * 230 Volts = 375 Watts. So to power this 200 Watt load would actually require a 400 Watt inverter.
This load looks awful, but it is in fact extremely common and is a typical load for just about every piece of equipment that uses some form of internal power supply. That includes TVs, PCs, DVD players, videos, radios, battery chargers, in fact just about everything you can think of barring a lightbulb or a heating element.
Because of the problems these sorts of loads cause to the national grid (higher peak currents and higher losses because these are proportional to I2) they have now been legislated against in Europe and it is expected the same will soon happen in most of the rest of the world.
However there is still a lot of this equipment in use and this will remain to be the case for a long time into the future.
All this means that a lot of modern equipment now has what is called "Active Power Factor Correction" (APFC) which causes the equipment to draw the current in a much cleaner (almost perfectly sinusoidal) manner which leads to lower peak currents and therefore smaller cabling, inverters, generators, isolation transformers etc.
This is a particular issue with high power (say above 20 amps) battery chargers which, if they have APFC, are able to run from much smaller generators than an equivalent charger without APFC.
And as in the previous cases of phase shift, measuring the voltage, measuring the current then multiplying them together will not give the Real Power. It will, again as in the previous case, give the apparent power. However in this case the Power Factor is rather more complex to calculate.
Most measuring instruments multiply the instantaneous voltage by the instantaneous current then average the result over a complete cycle. With distorted waveforms such as that shown above this really is the only reliable method.
And now you know just about everything you will ever need to know about Apparent Power, Real Power and Power Factor.
|
Page last updated 02/04/2008.
Website best viewed on a computer of some sort.